Viscoelastic beam Properties of a Lenticular Boom
In this example, we want to compute the Viscoelastic effective properties of a Lenticular Boom fabricated from plain weave composite material made of isotropic viscoelastic matrix and transversely isotropic elastic fiber. The MSG solid model is used to predict the effective viscoelastic properties of a plain weave composite using a three part approach.
The first part predicts the effective viscoelastic yarn properties based on the elastic fiber and viscoelastic matrix properties at the microscale. The second part takes the effective yarn properties and matrix properties to predict the viscoelastic properties of weave composites. The third part takes the effective weave properties to predict the viscoelastic properties of the Lenticular Boom.
Yarn
Weave
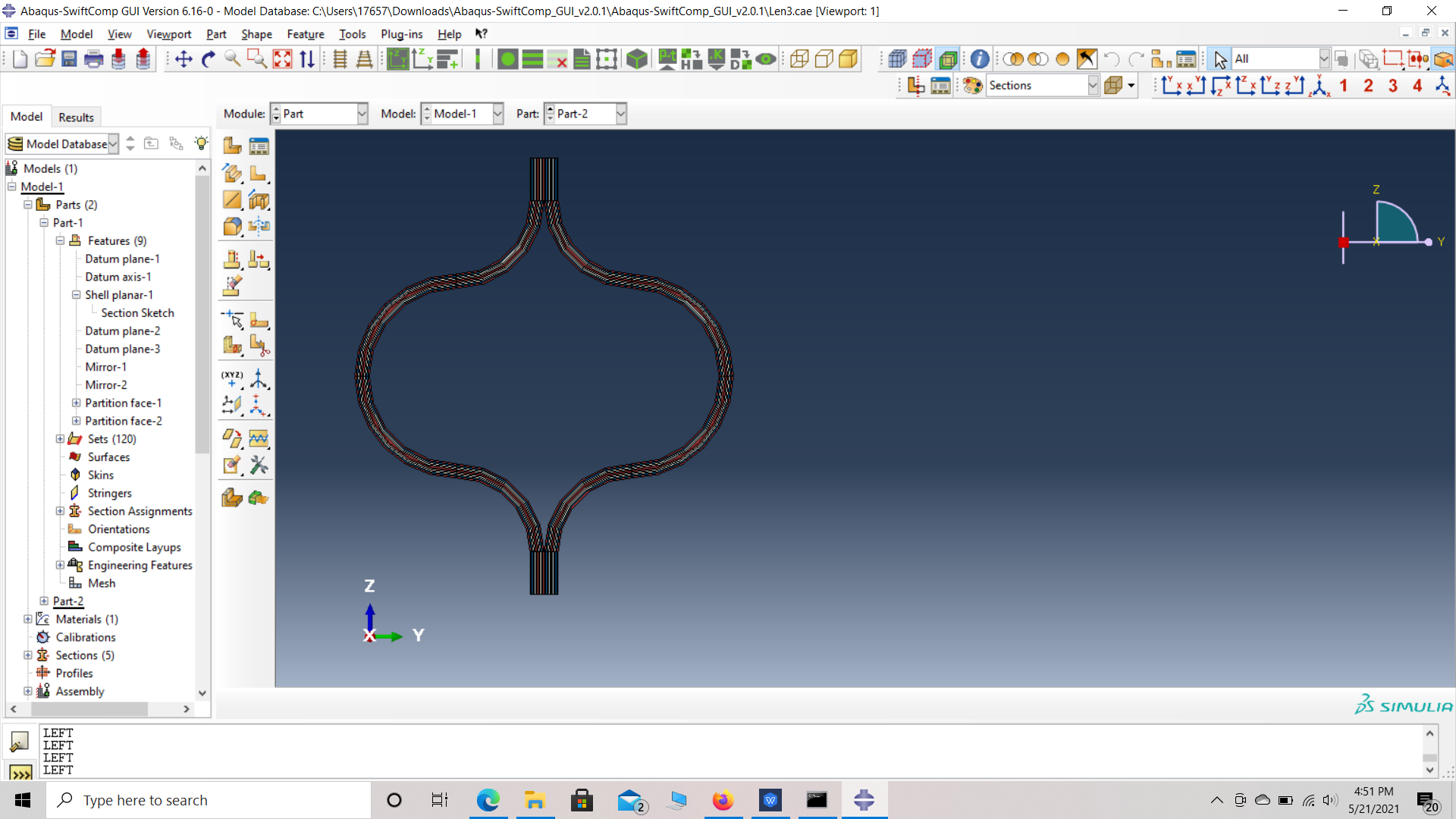
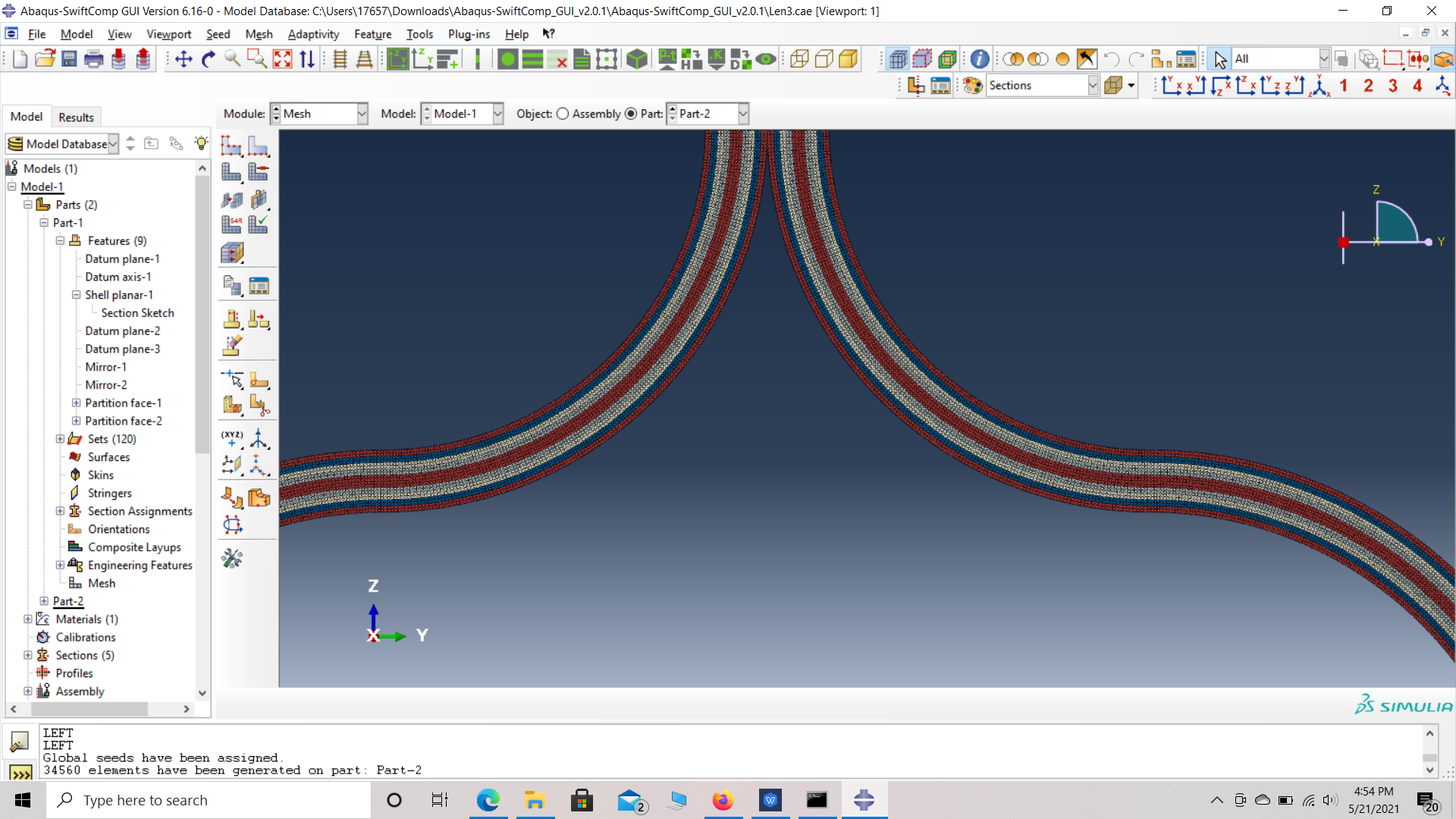
Lenticular boom
Web height: 3 mm, Subtended angle: 90 degree, Radius of the flange (curved part): 6.21 mm, flange thickness is 1 mm.
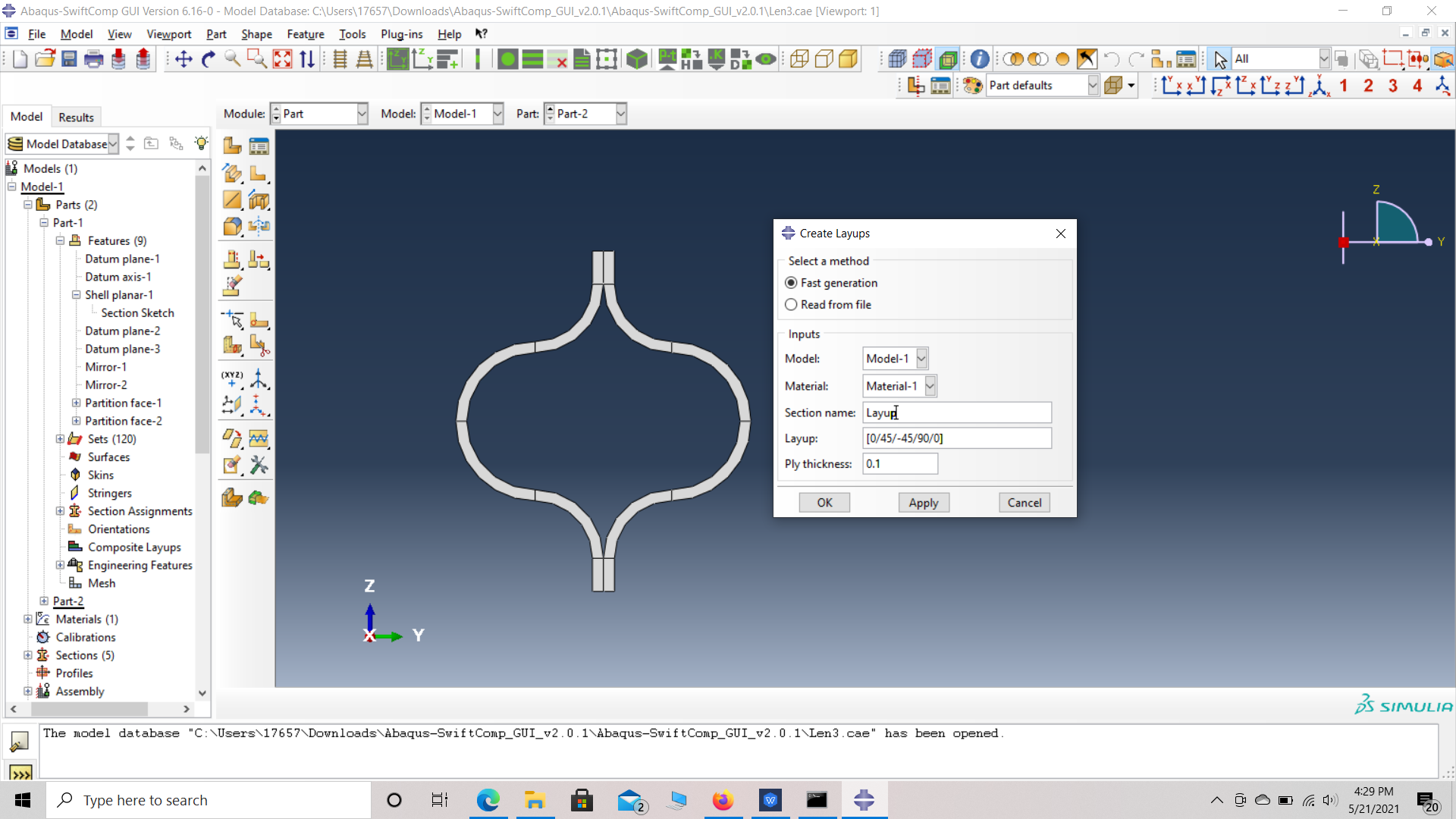
The Layup is (0/45/-45/90/0)s , Ply thickness: 0.1 mm. The layup sequence is along with the direction pointing outward from the center.
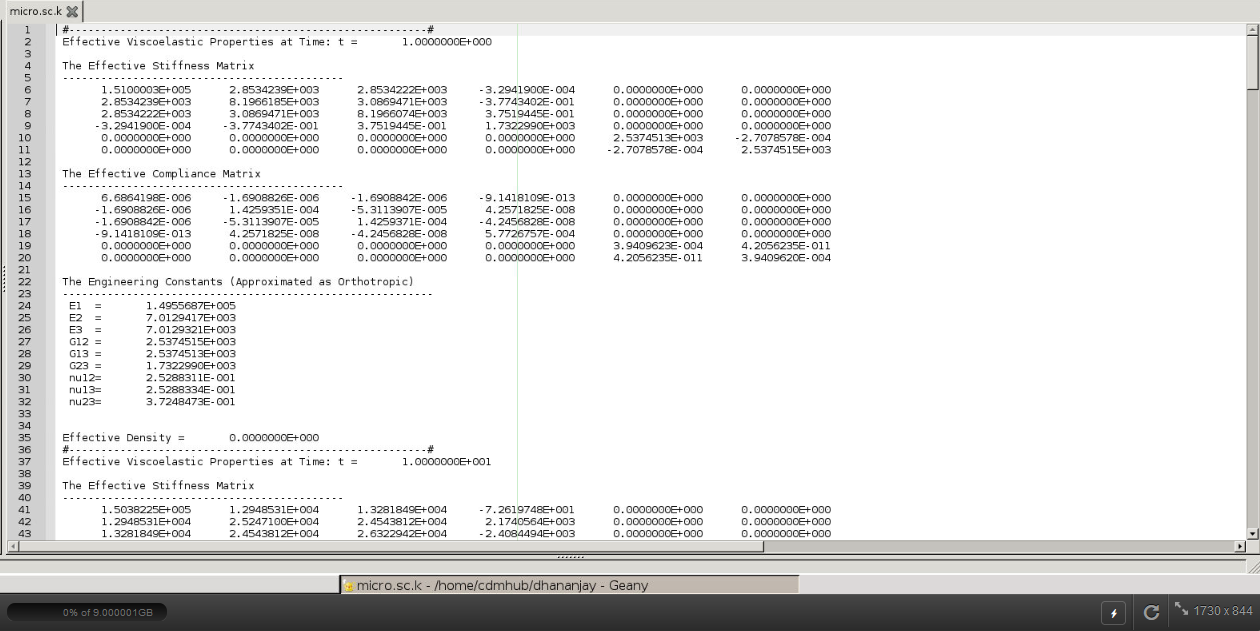
The fiber properties are defined as transversely isotropic elastic by means of engineering constants and the matrix properties are given as a time-dependent properties with a constant Poisson’s ratio equal to 0.33 as specified in the table below.
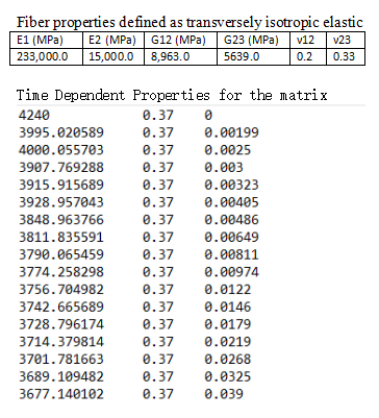Material Properties
The material properties should be defined as follows in different columns based on the type of material anisotropy. Each row corresponds to a different time interval.
– Transversely isotropic. —- Young’s Modulus, E (t) —- Poisson’s ratio, nu (t) —- Time, t
– Orthotropic defined by means of engineering constants. —- E1 (t) —- E2 (t) —- E3 (t) —- nu12 (t) —- nu13 (t) —- nu23 (t) —- G12 (t) —- G13 (t) —- G23 (t) —- Time, t
– Orthotropic defined by means of stiffness matrix. —- D1111 (t) —- D1122 (t) —- D2222 (t) —- D1133 (t) —- D2233 (t) —- D3333 (t) —- D1212 (t) —- D1313 (t) —- D2323 (t) —- Time, t
– Anisotropic. —- D1111 (t) —- D1122 (t) —- D2222 (t) —- D1133 (t) —- D2233 (t) —- D3333 (t) —- D1112 (t) —- D2212 (t) —- D3312 (t) —- D1212 (t) —- D1113 (t) —- D2213 (t) —- D3313 (t) —- D1213 (t) —- D1313 (t) —- D1123 (t) —- D2223 (t) —- D3323 (t) —- D1223 (t) —- D1323 (t) —— D2323 (t) — Time, t
We will use a square pack 2D SG with fiber volume fraction equal to vf = 0.64.
Software Used
We will use TexGen4SC 2.0, SwiftComp 2.1 and Abaqus CAE with the Abaqus SwiftComp GUI for this tutorial. TexGen4SC 2.0 will be used to run the viscoelastic homogenization of the fiber-matrix square pack microstructure and also for the viscoelastic homogenization of the plain weave laminate. Abaqus CAE will be used to model the boom and to run the viscoelastic homogenization while SwiftComp runs in the background.
Solution Procedure
The problem is solved in the following three steps:
Part 1- Micro-scale analysis of the square-pack fiber matrix micro structure using Texgen4SC.
Part 2- Meso-scale analysis of the plain weave laminate using Texgen4SC2.0.
Part 3- Macro-scale analysis of the lenticular Boom using Abaqus CAE with the Abaqus SwiftComp GUI and SwiftComp 2.1.
Part 1- Micro-scale analysis of the square-pack fiber matrix micro structure using Texgen4SC.
TexGen4SC 2.0 provides a function to let users import the material properties from a text file. Refer to the Predict viscoelastic plate properties of a single-layer plain weave laminate tutorials for more details regarding preparation of the materials text file.
Follow the step-by-step procedure to solve the problem.
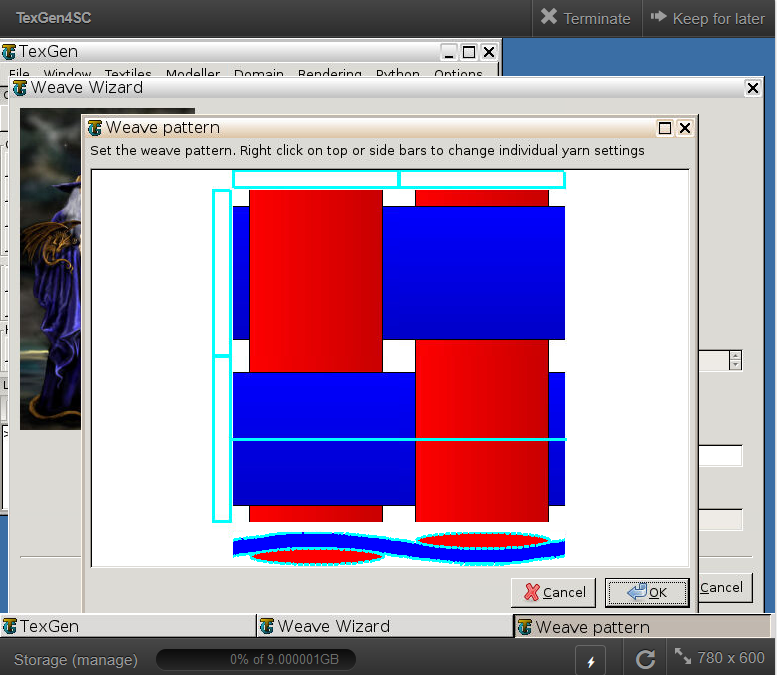
# Step 1.1. Create the plain weave pattern using TexGen4SC 2.0. Launch TexGen4SC 2.0 on cdmHUB, the Go to window-> controls-> “Weave” to create mesoscale plain weave SG.
Weave Wizard
# Step 1.2. Keeping the geometric properties as required, Click on the upper-right and lower-left squares to get the woven pattern.
Weave Pattern
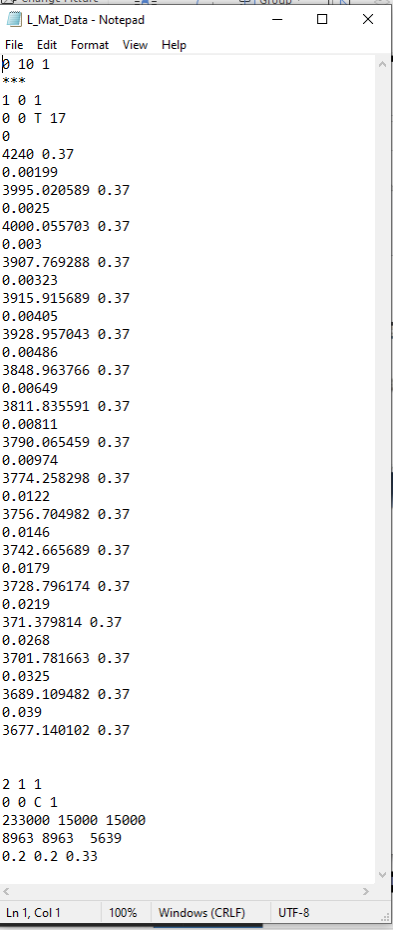
# Step 1.3.Upload the .txt file containing matrix and fiber properties to the current session, using any FTP app, for example, FileZilla, to set up connection with the current session. Refer to the Predict viscoelastic plate properties of a single-layer plain weave laminate Tutorials for more details.
Importing Material properties text file
Material properties text file
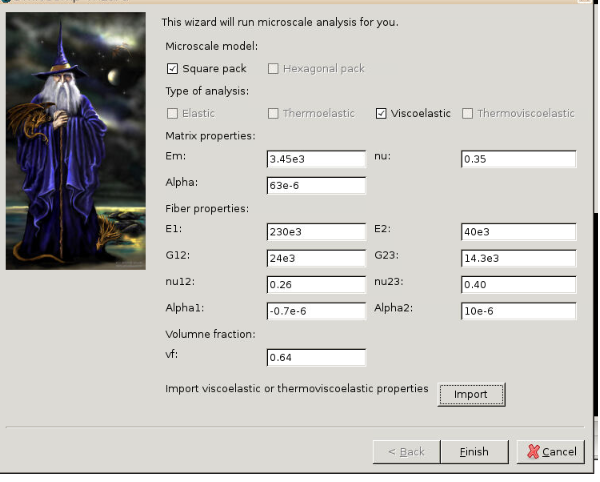
# Step 1.4. Once you uploaded the .txt file, click “Microscale” under “Homogenization” tab for yarn property calculation. Select “Viscoelastic” as the type of analysis. Set fiber Volume fraction as 0.64.
Homogenization
# Step 1.5. Ignore the matrix and fiber properties in the window, since the material properties will be imported from the uploaded file.
Property file
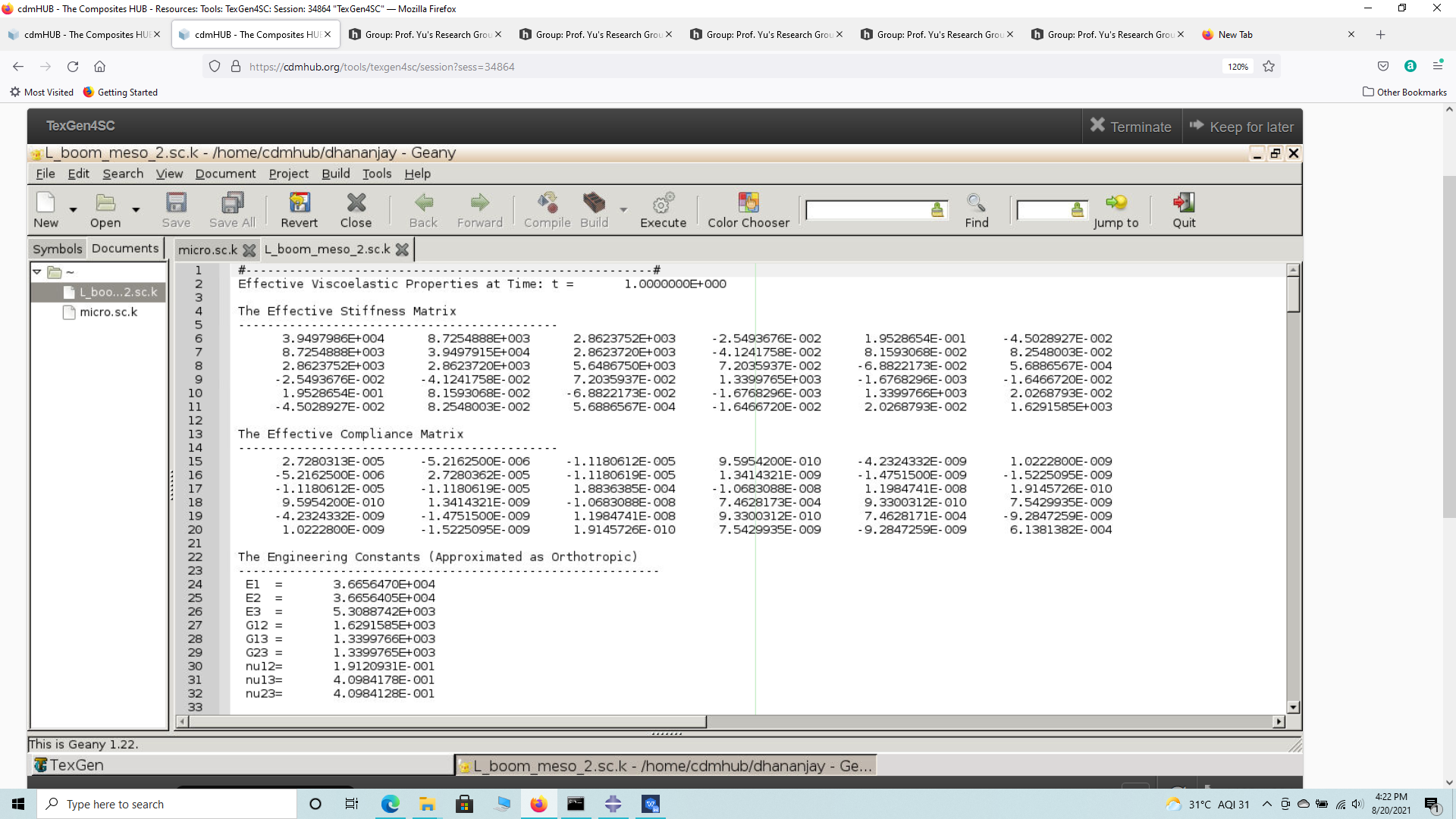
# Step 1.5. Click “Import” and select the uploaded .txt file and Click “Finish”. Now a .sc file (micro.sc) will be generated that SwiftComp will take as the input. SwiftComp will run on the cloud to calculate viscoelastic properties, e.g., effective microscale properties. In the pop-up window, you will find the analysis results.
Micro scaleResuts
Part 2- Meso-scale analysis of the plain weave laminate using Texgen4SC.
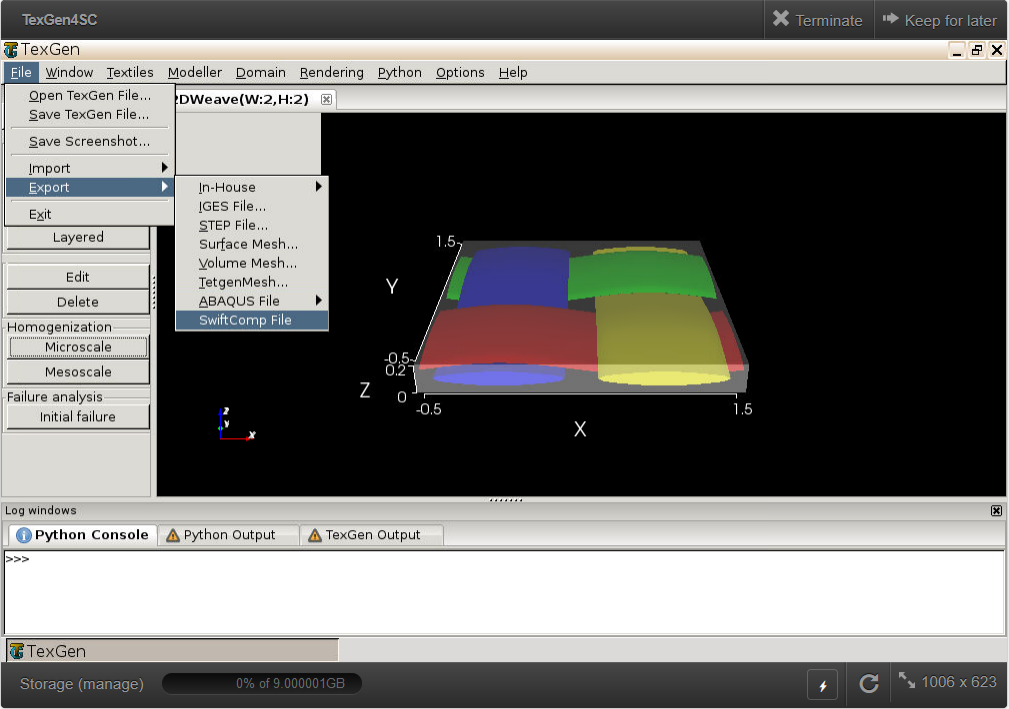
# Step 2.1. Go to “File->Export->SwiftComp File” to generate the .sc file for mesoscale analysis.
weave mesh
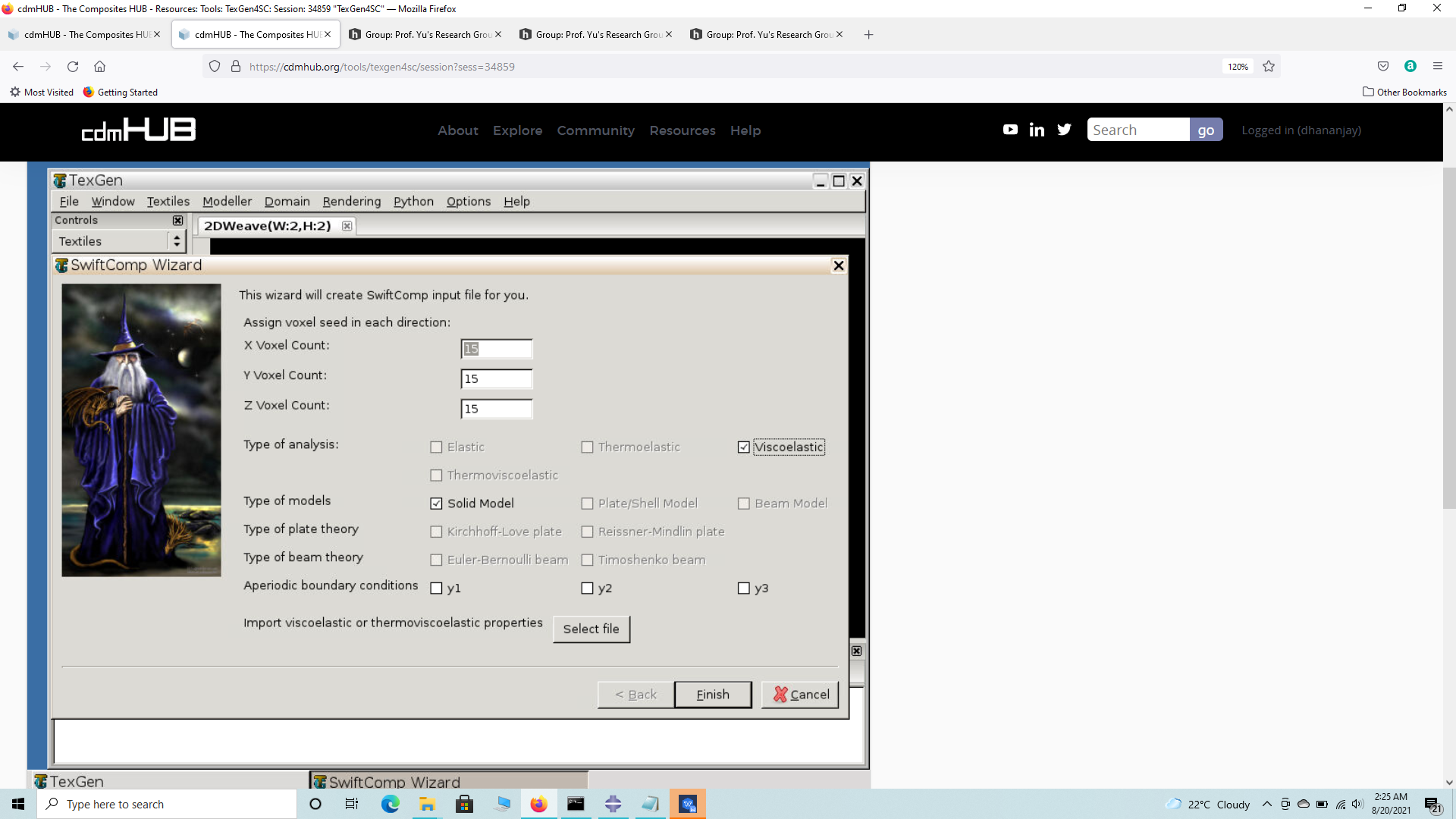
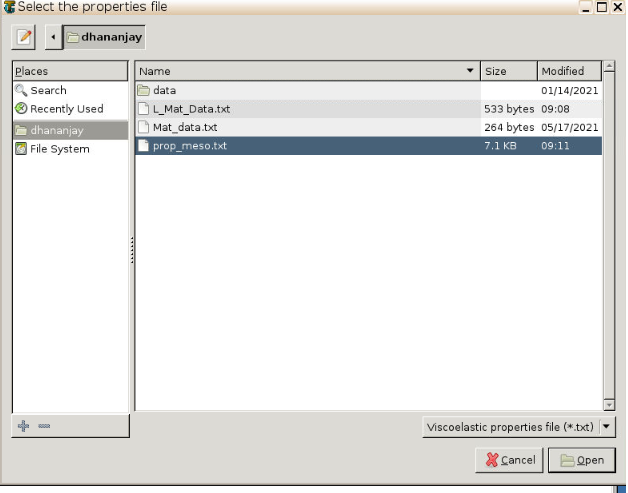
# Step 2.2. Define the voxel mesh, Select “Viscoelastic” as Type of analysis and Select “Solid model” . Click “Select file” and select “prop_meso.txt” which is automatically generated during microscale analysis, and will be used as part of mesoscale analysis input file.
SwiftComp Wizard
Property file
# Step 2.3. Save the .sc (SwiftComp input file) file with a filename of your choice. Click “Mesoscale” in “Homogenization” tab, which will call SwiftComp to calculate fabric properties.
Meso Scale Results
# Step 2.4.Transfer this file to your local computer for further analysis.
Part 3- Macro-scale analysis of the Lenticular Boom using Abaqus CAE with the Abaqus SwiftComp GUI and SwiftComp 2.1.
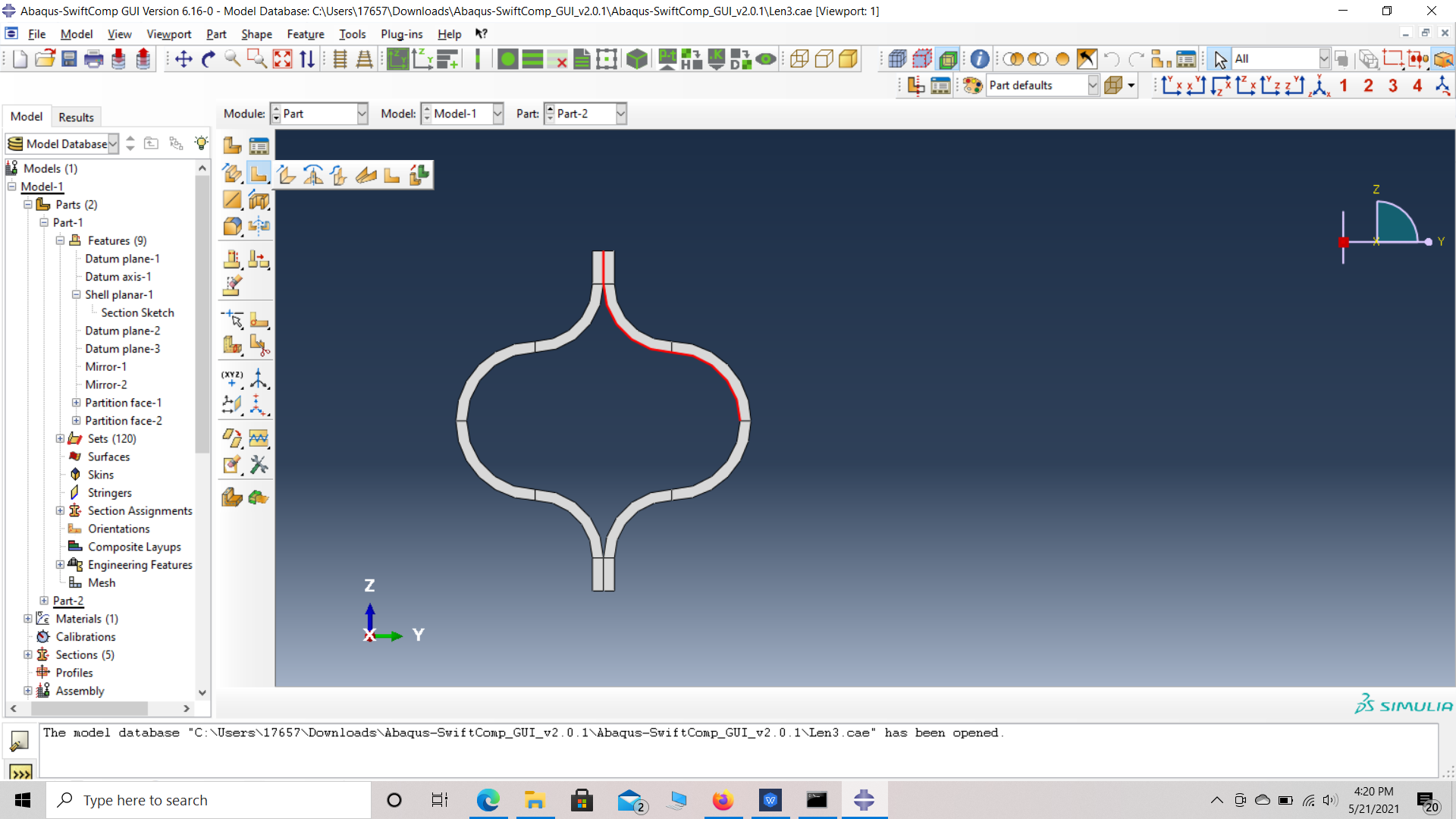
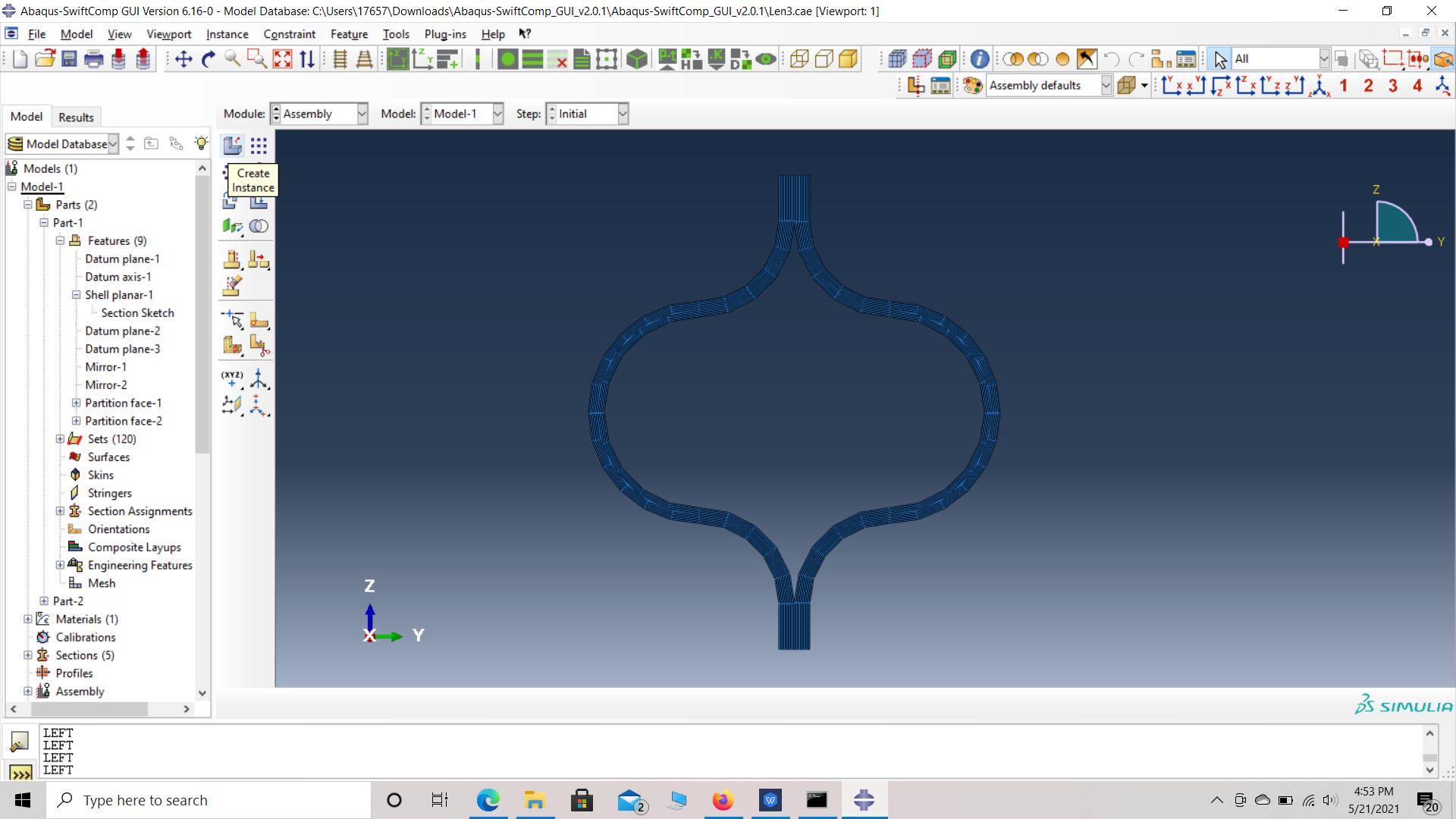
# Step 3.1. Using Abaqus CAE with the Abaqus SwiftComp GUI plugin, Create the part geometry for the Lenticular Boom. Use Set sketch plane for customized SG -> Create planar shell -> Select the plane and vertical axis -> Sketch a fourth of the base line (Highlighted as a red line). Its geometry is a straight line (Web height) from (0,12.42) to (0,15.42) and two curved lines (Flange) one from (0,12.42) to (6.21,6.21) centered at (6.21,12.42) and another from (6.21,6.21) to (12.42,0) centered at (6.21,0). Set its thickness to 1 mm to the right of the baseline by adding a straight line from (1,12.42) to (1,15.42) and two curved lines (Flange) one from (1,12.42) to (6.21,7.21) centered at (6.21,12.42) and another from (6.21,7.21) to (13.42,0) centered at (6.21,0). Close the section at its two ends and finish the part. Mirror the part about the vertical to get the geometry of the Boom. Partition the part to separate the web and flange and also the individual flanges.
Part Geometry
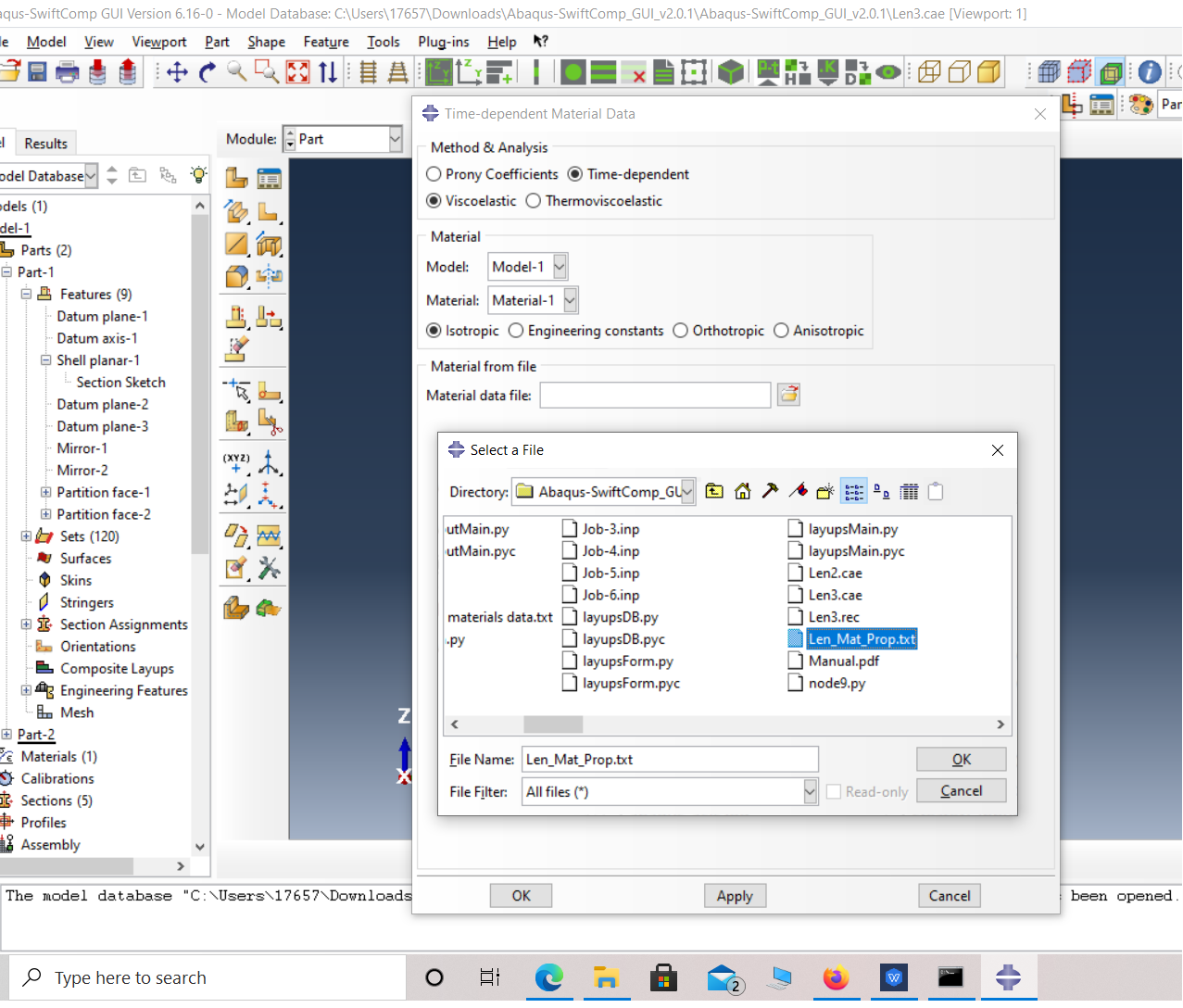
# Step 3.2. To enter the material properties for the part, first we need to choose the material properties from the results of the computed effective viscoelastic properties in the previous part. Within the Materials section of Abaqus CAE, we create a dummy material called “Material”. Please note that we will not define the material properties using the Abaqus SwiftComp GUI. Refer to the Computation of effective viscoelastic properties and time-dependent constituent properties with Abaqus SwiftComp GUI tutorial for more details.
dd
‘ # Step 3.3.Use the material properties from the previous section . We need to convert the Constitutive relations provided as SwiftComp’s results into Abaqus’s Constitutive relations. This can be done by switching the 4th and 6th rows for the relation and also switching the 4th and 6th column of the stiffness matrix. The relations are provided below. Since the material properties are given as a time-dependent properties, We will create a text file to input the time-dependent material properties described row wise as
– D1111 (t) —- D1122 (t) —- D2222 (t) —- D1133 (t) —- D2233 (t) —- D3333 (t) —- D1112 (t) —- D2212 (t) —- D3312 (t) —- D1212 (t) —- D1113 (t) —- D2213 (t) —- D3313 (t) —- D1213 (t) —- D1313 (t) —- D1123 (t) —- D2223 (t) —- D3323 (t) —- D1223 (t) —- D1323 (t) —— D2323 (t) — Time, t for all 17 time intervals.
Abaqus's Constitutive relations
SwiftComp's Constitutive relations
SwiftComp's output stiffness matrix converted into Abaqus's input stiffness matrix
(Image(L3.34.png, desc="Rearranged Material properties ") failed - File not found)
Material properties file
# Step 3.4. Now go to New Layups and add the material, section name, Layup and thickness to create the required layup. This can be repeated if we have multiple layups. We will use (0/45/90/-45/0)s as the laminate for the Lenticular Boom.
Layups
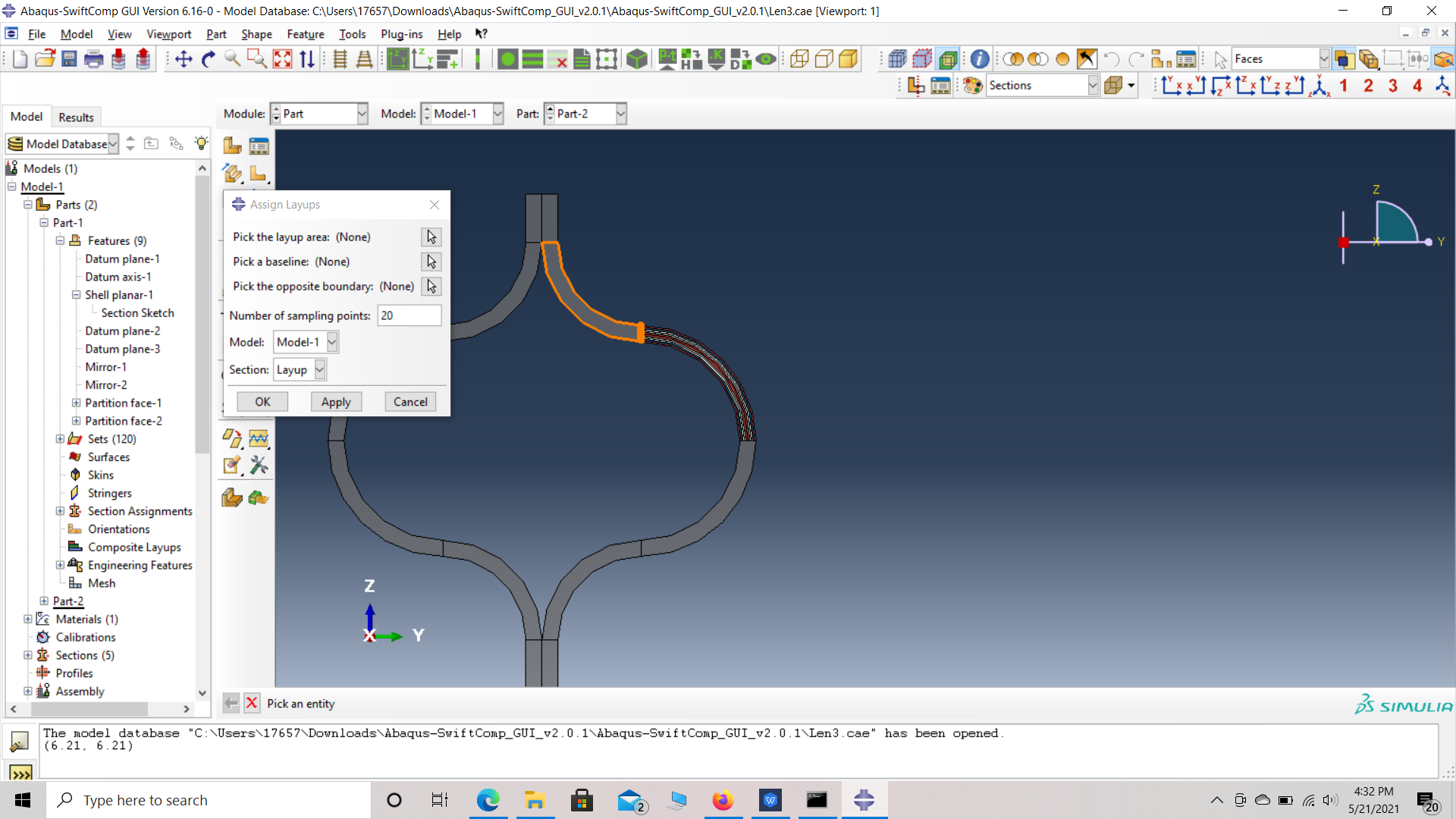
‘ # Step 3.5. To assign the layup, go to Create 2D SG: Assign Layups and the pick the baseline, the line opposite to the baseline and the area between the two picked line for the right flange as shown and then hit Ok. Do this for all twelve sections
Assign Layups
Assign Layups
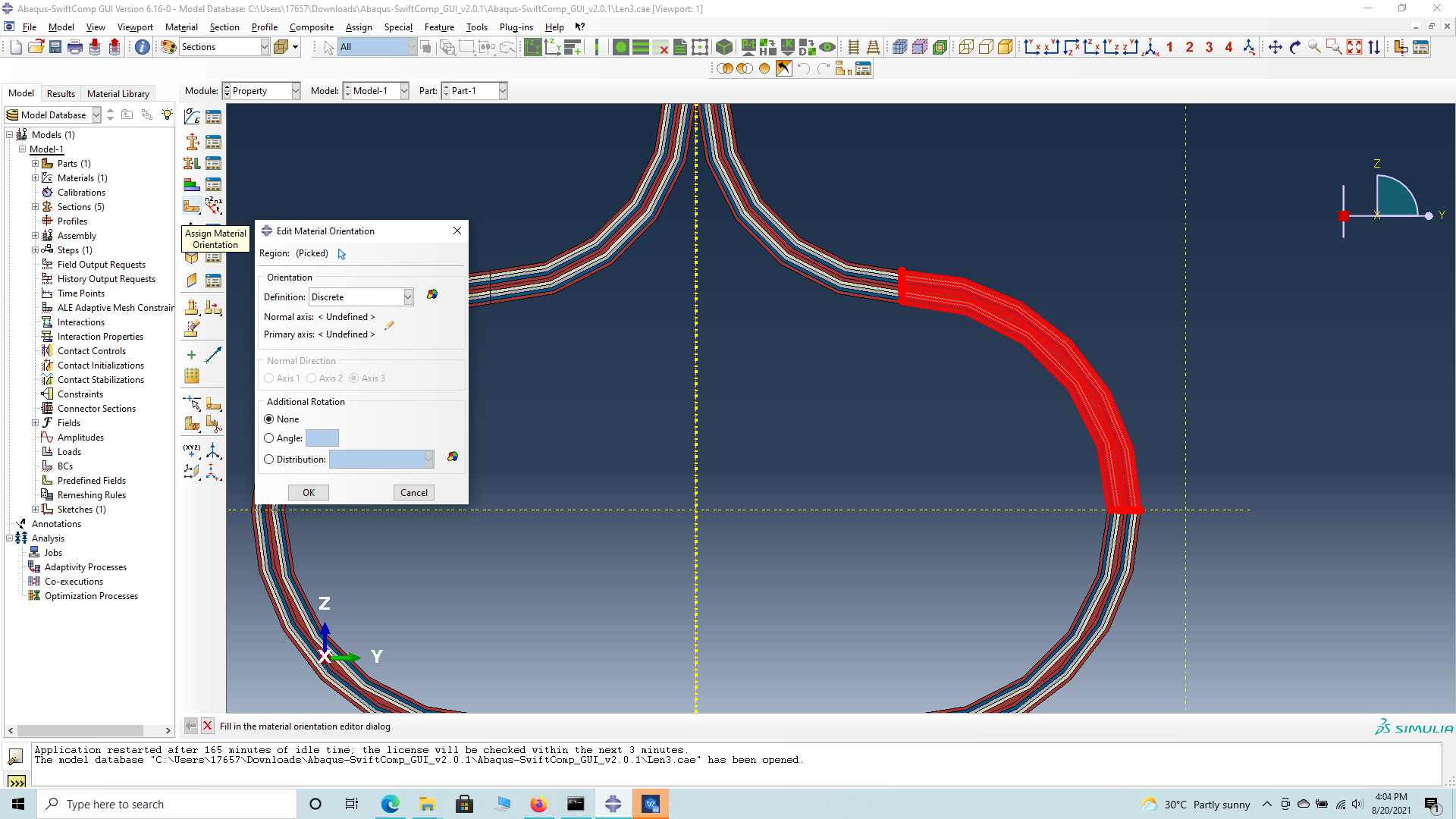
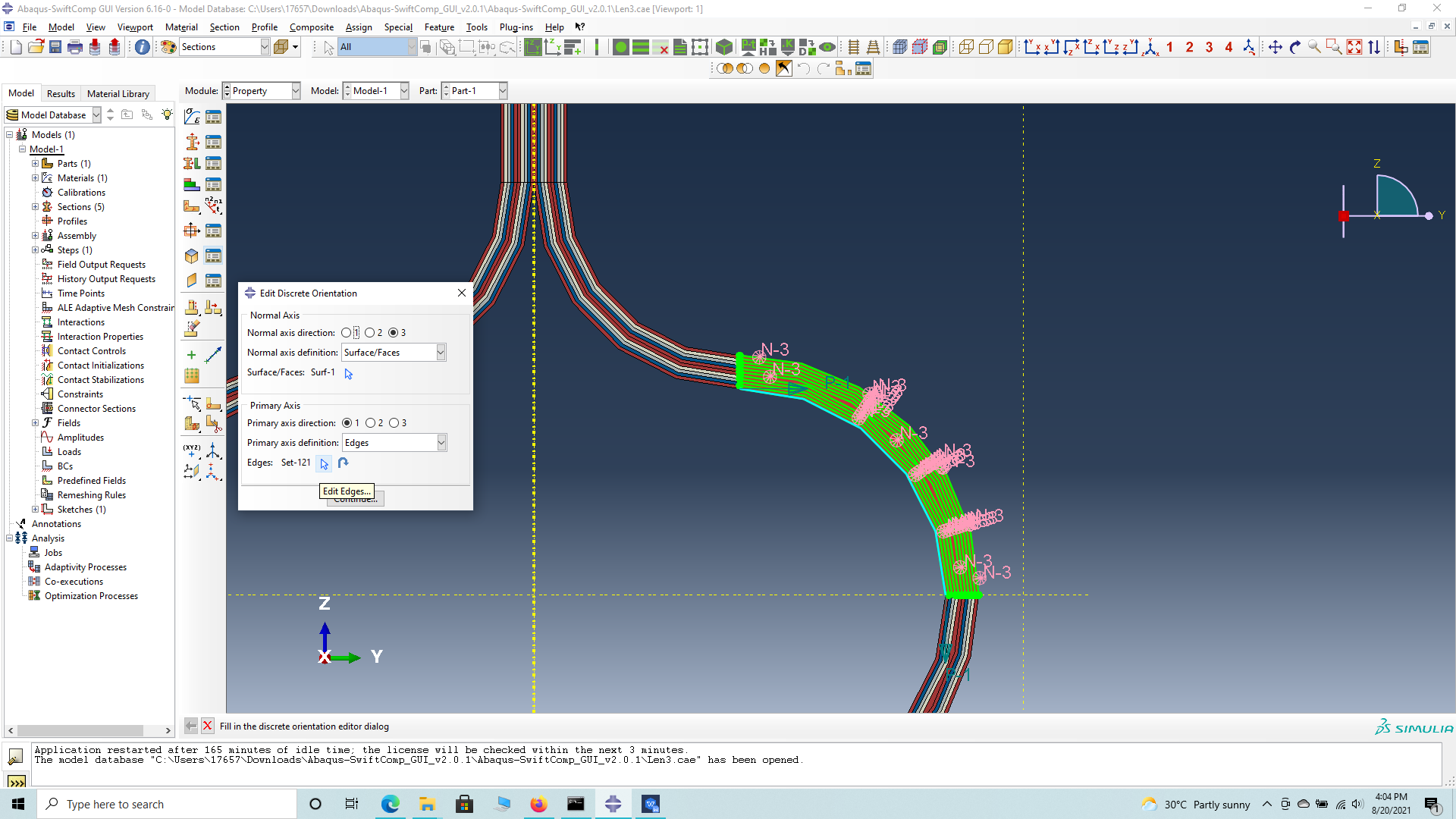
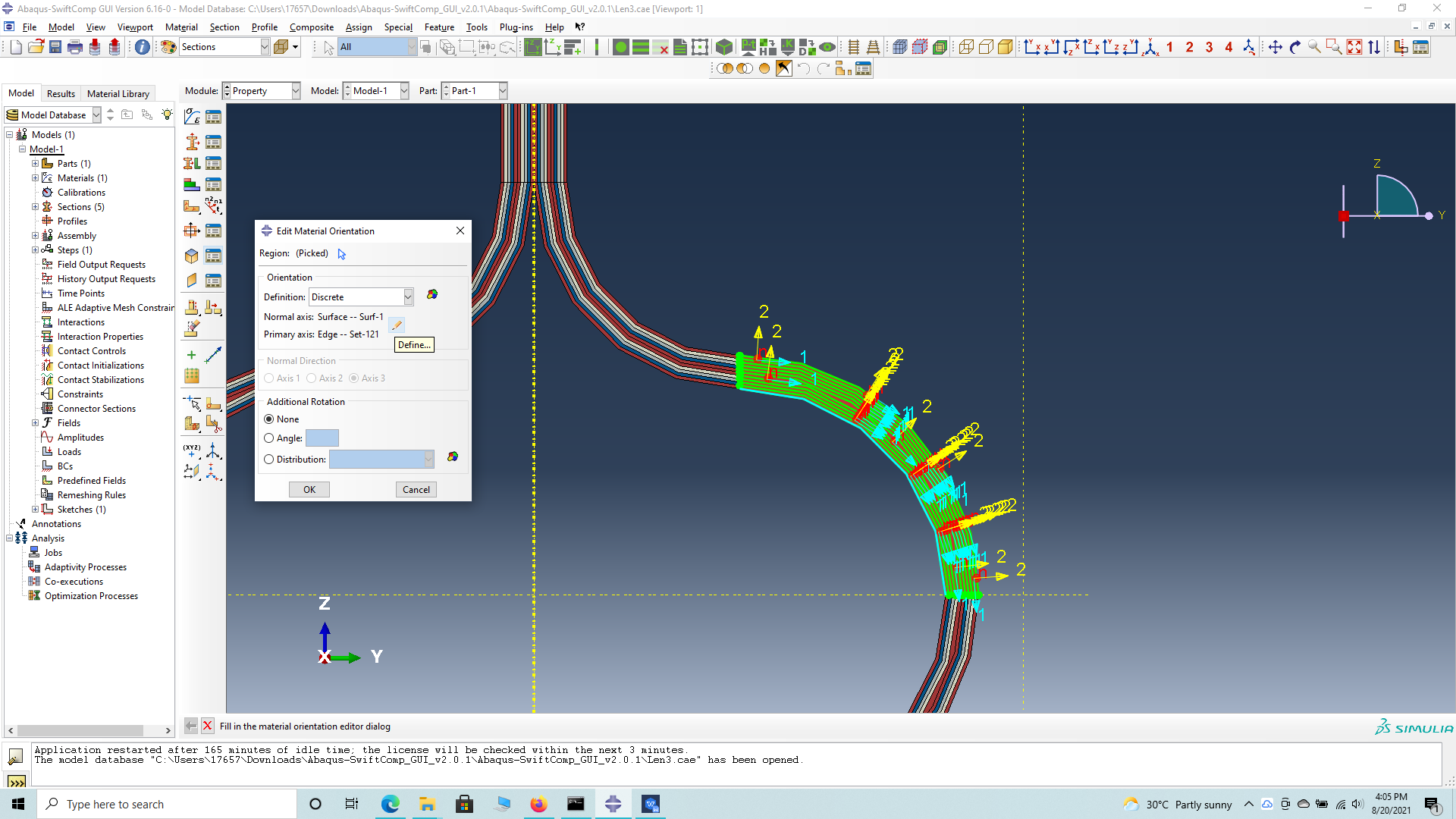
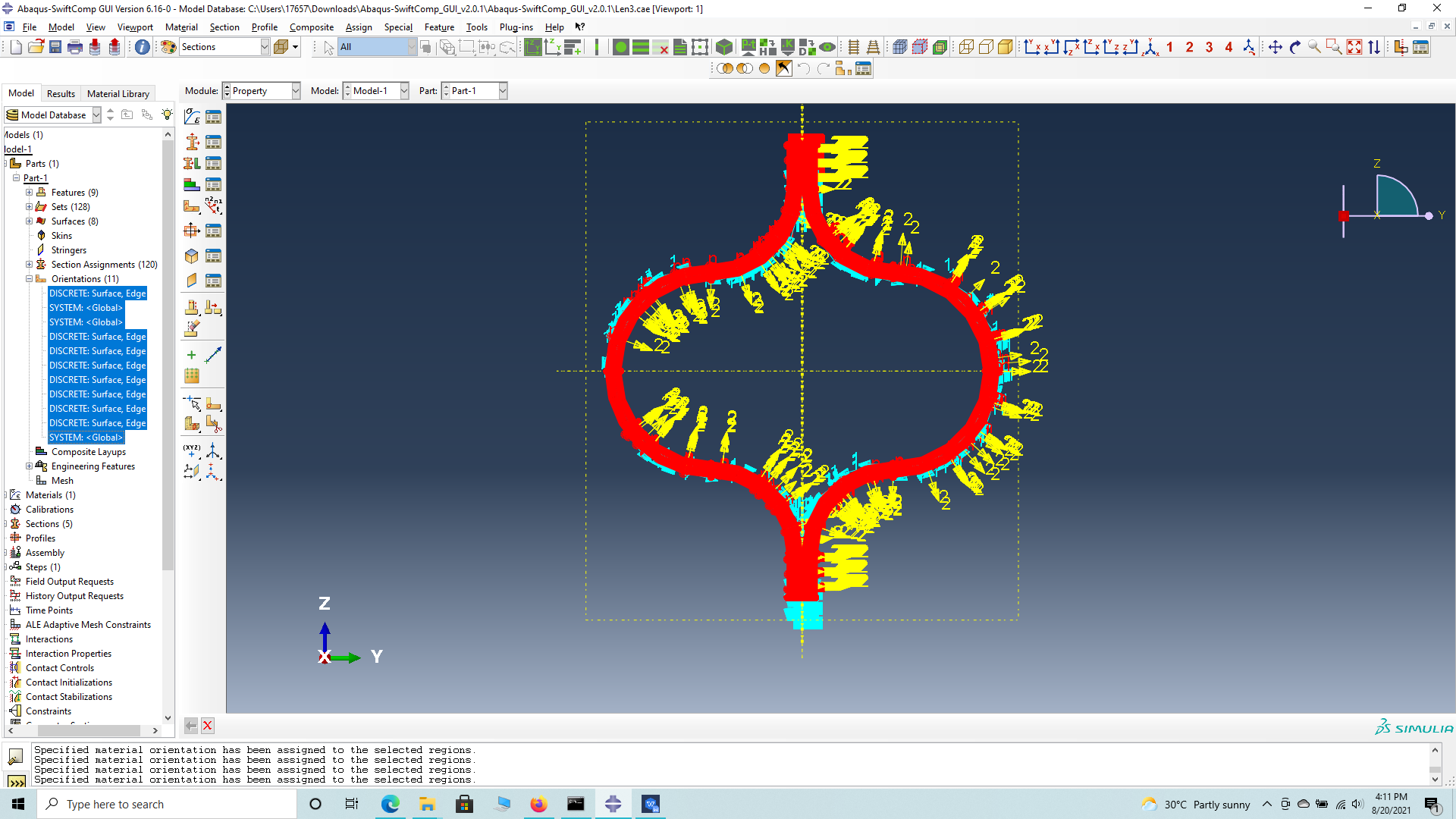
‘ # Step 3.6. Now we assign the material orientation for the part. Go to Assign material orientation -> select the sections of the part with the same orientation -> Done -> Select a CSYS (use default orientation or other method) -> Definition (Discrete) -> Define -> Primary axis orientation -> choose edge and flip direction if needed to make the axis point towards a clockwise direction -> Choose the surfaces for the normal axis definition -> Continue -> OK. Orientation Axis 1 represents the y2 axis of SwiftComp’s local orientation and orientation axis 2 represents y3 axis of SwiftComp’s local orientation.
Define material orientation
Edit edges
flange section orientation
part orientation
‘ # Step 3.7. Now go to Assemble, create the part instance with dependent mesh.
assembly
# Step 3.8.In the Mesh section, Seed the Part and set approximate global mesh size, then Click ‘Mesh Part’
Mesh
# Step 3.9.Create a job and write its input file.
ip file
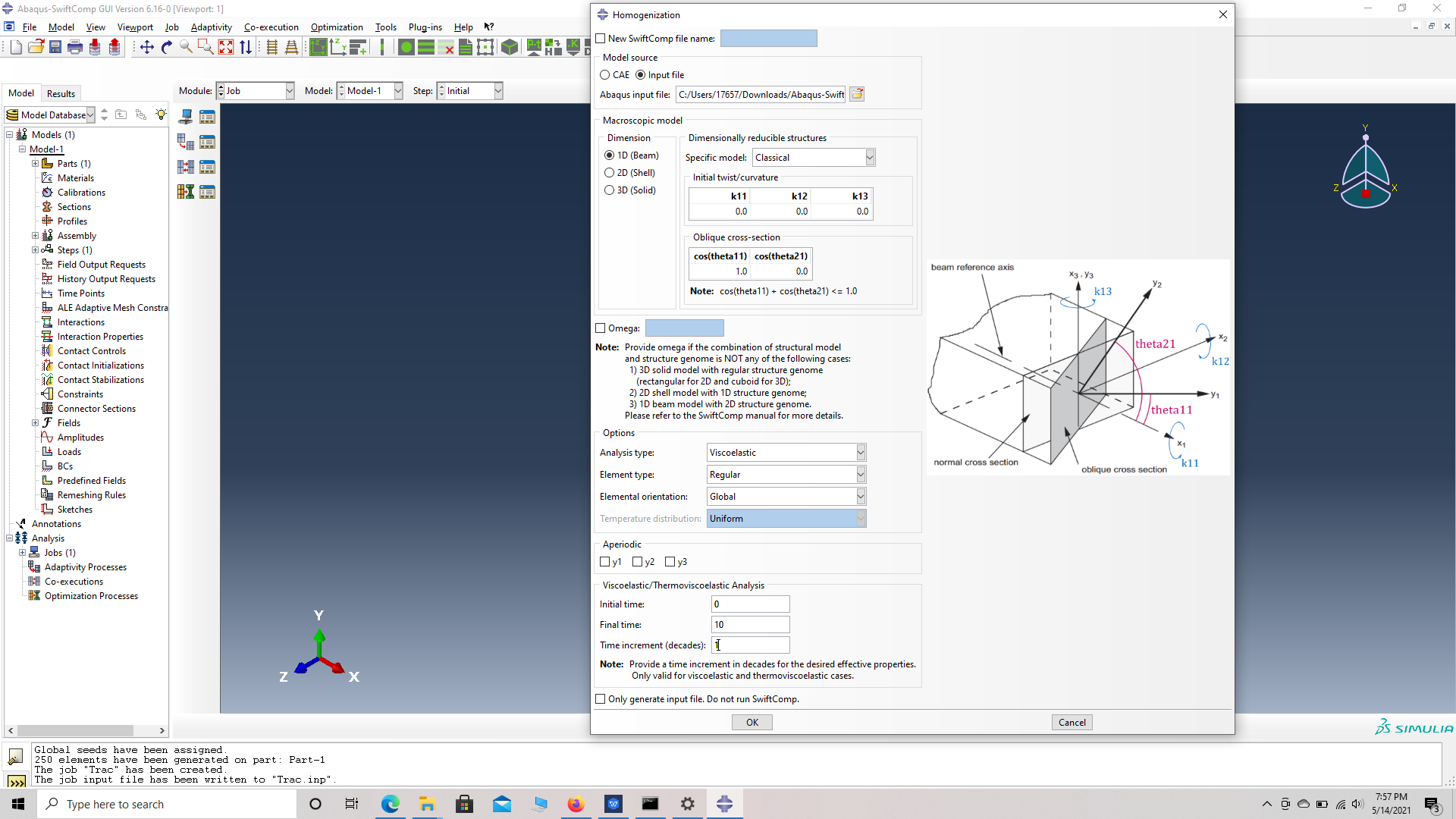
# Step 3.10.To the effective viscoelastic properties. we click on Homogenization and select Viscoelastic in Analysis Type. In the Viscoelastic/Thermoviscoelastic Analysis section, we define the range of the time (i.e. Initial time” and Final time”) in which we want to output the effective properties as well as the frequency (i.e. Time increment” defined in decades). Homogenize the part preferably as a beam using the Homogenization via input file option to get the final results.
Homogenization
References
# Rique, O.; Liu, X.; Yu, W., Pipes, R. B.: “Constitutive modeling for time- and temperature-dependent behavior of composites,” Composites Part B: Engineering, Vol 184, March 2020, DOI: 10.1016/j.compositesb.2019.107726.